click!
Sometimes you get something which suggests that it'll work ... like this picture:

Then again, sometimes it don't work.
| Date: Fri May 16, 2003 From: KenM |
For example, the S&P500 is at about the same value as 6 years ago in 1997. If, rounding-off, the SWR for 40 years is 4%, and I retired now I might say to myself that I'm only going to live 30 years so I'll withdraw a large lump sum equivalent to the total amount I would have withdrawn over 6 years if I'd retired in 1997, have a good time with all the money, and start annual withdrawals at 4+% (i.e. 4% plus 6 years of inflation). There must be something wrong somewhere.
| Date: Sat May 17, 2003 From: JWR1945 |
You are focusing on the logic behind statistically based projections. That is much more important than the projections themselves.
If you limit yourself to assuming that future sequences of investment returns are restrained exactly to those of the historical record, then you must come to a nonsense conclusion such as you have mentioned.
Have fun.
John R.
| Date: Sun May 18, 2003 From: gummy |
Hey! Neat idea! So why does randomness throw a monkey wrench into a perfectly logical suggestion, eh?
Like John R. suggests, ya gotta play with the randomness so here's a neato spreadsheet (if'n y'all got Excel):
click!
Sometimes you get something which suggests that it'll work ... like this picture:

Then again, sometimes it don't work.
| Date: Sun May 18, 2003 From: JWR1945 |
gummy tells us that:
Quote:
"Sometimes you get something which suggests that it'll work ... like this picture: "
All that you have to do is follow the same advice that Will Rogers is credited with giving for selecting stocks. Only buy those that
go up. If your stock doesn't go up, don't buy it in the first place.
Have fun.
John R.
| Date: Sun May 18, 2003 From: KenM |
I used the equal S&P500 values of 1997 and 2003 as a simple example. But if, starting withdrawals in 2003 at amounts to suit year 6 of a 40 year series starting in 1997, I still find it difficult to understand why I don't get the same probability of success for the period ending 2037.
I liked the Sam and Sally spreadsheet and noted that pressing F9 a lot of times indicated that Sam and Sally had very similar failure rates. So is there a free lunch after all?
I also note that the Sam and Sally projections follow the same path but that Sally's finishes 10 years earlier. Is it equally valid to say that Sally could start at year 10 of the Sam projection and follow Sam's path until year 40?
Didn't do very well with my kids' probability theory homework - gotta get in more practice for my grandchildren's.
| Date: Sun May 18, 2003 From: JWR1945 |
The probability of success does not scale directly with your portfolio's starting balance.
You are assuming that those withdrawals have a mathematical property called linearity. That is, you are assuming that the portfolio balance decreases by a fixed number of dollars per year. If that were true, a shift in your starting balance would result in a comparable shift in the lifespan of your retirement portfolio. Double your starting balance and you double its lifespan.
Picture two different graphs of a portfolio balance versus time. In the first graph, the balance remains very high until the last two or three years. In the second, the balance drops dramatically at first and then declines very slowly...almost remaining constant.
Those two cases are plausible enough albeit artificial. They correspond to having steady returns over a very long period of time...along with a devastating bear market or crash either late or early.
In the first instance you could have started out with a low starting balance and done very well for thirty seven years. If you tried to do the same thing in the second example, you would have been wiped out immediately by the crash (or by the bear market). In neither case does your portfolio lifespan scale directly with your starting balance.
| Date: Sun May 18, 2003 From: JWR1945 |
KenM is thinking along the lines of tossing a coin, specifically a hypothetical fair coin. The coin has no memory of the past. The probability of heads is the same with each toss. The outcome of each toss is independent of the previous tosses.
From one year to the next, stock price fluctuations are reasonably close to being entirely random...to being truly independent. But if you look at long intervals of time, that is no longer true. That is one of the reasons that raddr's discovery that mean reversion exists (along with his precise definition of that term) is so important.
Although stock prices may seem to fluctuate randomly from one year to the next, inflation does not. That was one of gummy's findings. His treatment of inflation is a highly significant feature of his Monte Carlo safe withdrawal rate calculator.
The actual history of the years from 1997 to 2003 have filtered out many of the possibilities for the period from 2003 to 2037. That fact that the bubble has burst means that we are less likely to see a comparable bubble in the near future.
In terms of KenM's attempts at doing this:
Quote:
"John doubts I'm a statistical idiot - I always like to prove I'm right."
He is doing poorly.
Have fun.
John R.
| Date: Mon May 19, 2003 From: gummy |
Overheard at the local coffee shop:
Sam: I'll live for 40 years and need $40K per year and a 4% withdrawal from my $1M portfolio is safe so I'm happy.
Sally: I'll also need $40K per year from my $1M portfolio. But I'll live for just 30 years, so I'll take out 10 years worth of $40K - that's $400K - leaving me with $600K. I'll spend the $400K on fun-and-games and withdraw $40K per year from what's left - that's the $600K.
Sam: But you're withdrawing $40K from a $600K portfolio, not a $1M portfolio!
That's a withdrawal rate of, let's see: 40/6 = 6.7%. You think this is safe? You're dreaming!
Sally: Well, suppose I take out 5 years, that's 5 x $40K for fun & games. That leaves me with $800K. How's that?
Sam: You'll be withdrawing $40K from an $800K portfolio, that's ...
Sally: I can do the math! That's 40/8 or 5%. Is that safe, for just 30 years?
Sam: I doubt it ... even for 30 years.
Sally: How many years can I take out ... to play with?
Sam: If you want $40K per year and you take out N year's worth, that's N x $40K, then, let's see ...
Sally: I can do the math! What's left in my portfolio is $1M - $40K*N and I'm withdrawing $40K per year from this so ...
Sam: That's a withdrawal rate of 40K/(1M - 40K*N) or 40/(1000-40N) or, as a percentage:
That's 4000/(1000-40N)%.
Sally: I don't know if I agree with your formula. Let me check:
For N = 10 years that's 4000/(600) = 6.7% and for N = 5 years it's 4000/(800) = 5%. Yes, that's what I got above, so I agree.
Sam: Suppose you think x% is safe, then you'll want 4000/(1000-40N) = x so N = 25 - 1000/x meaning that ...
Sally: I can do the math! I think maybe 4.5% is safe, for my 30 years.
So I should be able to take out N = 25 - 100/4.5 = 2.8 years worth. What! That's all? I drop dead 10 years earlier yet I only get to play with ...
Sam: I suggest you live for 40 years.
| Date: Mon May 19, 2003 From: KenM |
Sam: 10 years ago I decided I'll live for 40 years and my friend Mr Monte Carlo told me that withdrawing $40,000 per year adjusted for inflation from my $1M portfolio would be 99% safe.
Sally: That sounds a great idea. Do you think I could do that starting now?
Sam: Well, after 10 years of withdrawals, my next one should be $55,000 but the market isn't too good right now and my portfolio's only worth $800,000. However I trust Mr MC and as he told me at the beginning of the 40 years that I would be 99% safe, I intend to take the full $55K.
Sally: That sounds even better, I really like your friend Mr MC . I only expect to live for another 30 years and my portfolio's coincidentally worth the same as your current $800,000. So I'll start withdrawing $55,000 now and still have the same 99% safety as you for the next 30 years.
Sam: But that doesn't seem fair. My initial withdrawal rate was 4%. Yours will be 6.9%. I'd better get Mr MC to buy me a free lunch.
| Date: Mon May 19, 2003 From: gummy |
It must be these aging grey cells 'cause I never quite understood what you were saying until your Sam & Sally story. (I love those stories. I have a few on my website. Usually Sally is the wiser. With the latest, I expect complaints from the gals ...)
I've put your pub scenario
here:
I hope you don't mind. (If so, I'll remove it.)
| Date: Mon May 19, 2003 From: KenM |
However, it may be there under false pretences. I still can't convince my own aging grey cells that Sally is wrong.
| Date: Tue May 20, 2003 From: gummy |
If Sally adopts Sam's portfolio/withdrawals at the 10-year mark and he's one of the 99% of those whose portfolios survive 40 years, then hers is guaranteed to survive the remaining 30 years.
The latest mods (to the tutorial) also provide an argument to show that Sally's withdrawal rate can be much higher than Sam's "safe" 4% ... or much lower 
However, my first understanding of your problem was that she didn't adopt his portfolio but just removed 10 of his annual withdrawals and started withdrawing from that reduced portfolio.
Anyway, thanks for an interesting problem!
P.S. You'll recognize the name attached to this spreadsheet, eh? KenM
| Date: Tue May 20, 2003 From: wanderer |
she's not... if the future is identical to the past. If, however, she has returns sequenced one year differently, she may be in trouble. see raddr's post for the sensitivity of "safe" withdrawal analysis.
gummy/kenm - funny, interesting stuff.
| Date: Tue May 20, 2003 From: KenM |
And hopefully gummy won't mind if I continue to use his Sam/Sally format (hope it's not copyright) - it definitely helps to define a problem and my own previous explanations were obviously not clear - and it makes Sam look stupid instead of me - I've tried to make Sally look the sensible one .
So, continuing the story in the pub:
Sam: 10 years ago I decided I'll live for 40 years and my friend Mr Monte Carlo told me that withdrawing $40,000 per year adjusted for inflation from my $1M portfolio would be 99% safe.
Sally: That sounds a great idea. Do you think I could do that starting now?
Sam: Well, after 10 years of withdrawals, my next one should be $55,000 but the market isn't too good right now and my portfolio's only worth $800,000. However I trust Mr MC and as he told me at the beginning of the 40 years that I would be 99% safe, I intend to take the full $55K.
Sally: That sounds even better, I really like your friend Mr MC . I only expect to live for another 30 years and my portfolio's coincidentally worth the same as your current $800,000. So I'll start withdrawing $55,000 now and still have the same 99% safety as you for the next 30 years.
Sam: But that doesn't seem fair. My initial withdrawal rate was 4%. Yours will be 6.9%. I'd better get Mr MC to buy me a free lunch.
Mr MC overhears the conversation
Mr MC: Sorry, no free lunches for anybody. I have to tell you Sally that your starting portfolio value being equal to Sam's is irrelevant to an SWR for the next 30 years. If you start at a 6.9% withdrawal rate at this point in time then you're only about 90% safe for a 30 year period. If you want 99% safety my calculations show that you'd better start at about 4.5%.
Sally: That's disappointing. I'll have to buy my own lunch.
Sam: But what about me? I'm now at a 6.9% rate and I need another 30 years the same as Sally. You promised me when I started that I would be 99% safe for 40 years. Are you telling me now that after 10 years I'm only 90% safe?
Mr MC: To put it bluntly, at this point in time, yes. I must have forgotten to tell you that if, at any time during your 40 years, the withdrawal rate exceeds the SWR for the remaining period of the 40 years, then your safety rate declines accordingly. I'd have thought that commonsense would have told you that.
Sam: But I'm an average investor, I don't have any commonsense. And anyway, Mr MC, all your calculations look so scientific and precise that people like me always believe what you tell us. So what are you telling me now so I don't misunderstand again?
Mr MC: For 99% safety over the whole period; with 40 years to go, your SWR is 4%, with 30 years left 4.5%, 20 years 5%, 10 years 6%. This is not precise, but if your portfolio value falls such that, at around those points in time, your actual withdrawal rate substantially exceeds the relevant SWR, then you don't have 99% safety of reaching the end of your 40 years.
Sam: I don't really like the sound of that. C'mon Sally, lets go and see that if that nice financial adviser will buy us lunch. He promises 8% withdrawal if we pay him a 1.5% fee.
That's how I understand the practical application of monte carlo simulations and resulting SWR's to an actual period of retirement. (Note that the quoted SWR's are guessed at/rounded off for illustrative purposes only). I'd be quite happy if somebody told me I'm wrong.
| Date: Wed May 21, 2003 From: gummy |
Anyway, here's my understanding:
According to Mr. MC, there's a 99% chance of Sam's $1M portfolio lasting 40 years. That means that, of 1000 40-year MC simulations, 990 will survive (assuming the future is like the past).
If Sally jumps in at the 10-year mark, then her portfolio is identical to his (for the last 30 years) ... hence there's a 99% probability that hers is one of those 990 surviving portfolios.
Of the 990 surviving portfolios, there will be plenty whose withdrawal rate, at the 10-year mark (as a percentage of the 10-year portfolio value), will NOT be the "MC safe" rate for 30 years ... some may be Sally's 6.9%.
That poses interesting questions:
Consider a jillion $1M portfolios over 40-years with a 4% withdrawal rate (where 99% of them survive).
Now look at these jillion portfolios at the 10 year mark.
(Some are $5M, some are $500K and, sadly, some are $0.)
Look at the withdrawal amount $A, at the 10-year mark.
(This is the same for all jillion portfolios if we assume a fixed inflation rate.)
For each of the jillion portfolios, we calculate $A/$Portfolio, the withdrawal rate at the 10-year mark.
Look at the Distribution of these withdrawal rates (at this 10-year mark).
Some will be large, some small ... and some will be Sally's 6.9%!
Question #1: What fraction of the jillion 10-year portfolios will survive another 30-years?
If one does MC simulations, then this Question might have an answer like 90% (adopting KenM's number) 
But we've assumed that 99% of the jillion portflios survive 40 years!
So how come only 90% survive the last 30 years?
Now forget all about the jillion portfolios and calculate the 30-year "MC safe" rate ... say 4.5% (adopting KenM's number)
Question #2: How does the 4.5% "MC safe" rate for 30 years compare to the Distribution (at the 10-year mark)?
Nice questions, eh? Now I'll go away and think ...
| Date: Wed May 21, 2003 From: JWR1945 |
Sam does not continue to be 99% safe. If his portfolio has gone up, his level of safety has increased. His first ten years were lucky. If his first ten years were unlucky, his portfolio has gone down. His chances of success have decreased.
The first ten years for Sam are now part of history.
Another way of looking at this is to ask the question, "How much should Sally invest?" When Sam started out, he had a wide range of possible portfolios balances at the ten year mark. Now, Sally has chosen only a narrow portion of those possible balances. At this point we begin to get very interested in the details of probability distributions.
Have fun.
John R.
| Date: Wed May 21, 2003 From:galagan |
JWR1945 -
I don't mean to be obtuse or nitpicky here, but this raises a question for me. My hope is that the question may help me understand better the effect of mean reversion-based simulations.
I understand that in general, the more money you have, the more likely it is that you'll be able to sustain fixed withdrawals for a fixed period of time. However, it seems to me that you are proposing two variables:
1. value of portfolio, and
2. level of asset valuations.
These two variables will generally have a positive correlation - that is, if valuations are relatively high, the value of one's portfolio will be relatively high as well.
So if Sam's history was bad in his first 10 years, one might assume that valuations were more reasonable after 10 years than at the beginning. These lower valuations might support a higher safe withdrawal rate. Whether or not the effect of the lower valuations outweighs the actual reduction of the portfolio is unclear. But I could imagine a possible result where a $1,000,000 portfolio fell to $800,000, but a 4 percent SWR rose to 5 percent as a result of more favorable valuations, which would lead one to conclude that Sam is in a relatively similar position to where he started despite the unfavorable history.
One could reverse this and argue that even though portfolio value increases, higher valuations more than compensate by increasing risk.
dan
| Date: Wed May 21, 2003 From: WaltDardenne |
Having read the Sam/Sally exchanges this morning, I couldn't get through breakfast without running a little trial:
Assume that Sam is retiring with $100,000 and knows that he'll live 5 years. He has access to Bill Bernstein's eccentric Uncle Fred's investment vehicle. At the end of each year, Uncle Fred tosses a coin. If it's HEADS, you get +30% return. If it's TAILS, you lose 10%. The average return is +10% and the geometric return is 8.17%.
Sam wants 90%+ success rate. Running through the 16 possible combinations of outcomes over the next 4 years, one can calculate that Sam's maximum withdrawal rate should be $17,323. This gives him a probability of success of 93.75%. (The above assumes he makes an immediate withdrawal and then 4 equal withdrawals in the future.)
Now, year 1 is over and we have the coin toss. If the toss is HEADS, then Sam has a new balance of $107,480 (before withdrawal) and his future successs rate is 100% if he maintains the initial withdrawal amount. If the toss is HEADS, then Sam has a new balance of $74,409 (before withdrawal) and his future successs rate is 87.5% if he maintains the initial withdrawal amount.
What should Sam do? What should Sally do if she now wants to retire for the remaining 4 years using Sam's strategy?
I'd say they should both recalculate to move the probablility of succes back towards 90%. If the toss was HEADS, Sam can increase his withdrawal to $22,783. If the toss was TAILS, Sam can decrease his withdrawal to $15,773. IMO Sally should follow Sam's lead and use an equivalent 20.2% withdrawal rate. They should both recalculate after each year's coin toss.
Obviously, this is a simple case and doesn't address mean reversion, etc. But, hopefully it is helpful. It was to me.
| Date: Wed May 21, 2003 From: JWR1945 |
Actually, the effect that you mention is true in general. It is not at all limited to specific models.
This effect involves conditional probabilities. In general, the overall probability that something will happen is the sum of each (conditional probability [each of which is restricted by the occurrence of a specific event]) * (the probability that the specific event happens). Every event has to be completely independent of the others (i.e., no overlap) and total number of events must cover all possibilities.
For example, if we declare failure as soon as a portfolio balance has fallen to zero, we can separate all of the possible portfolio balances at year ten into three groups (actually, three sets): balances that (have failed previously or currently) range from zero to $800K, balances between $800K and $1200K (i.e., from $800 000.01 to 1199 999.99) and balances of $1200K and above. We can then look at different withdrawal rates at the beginning of a 40-year period and see how many (what percentage of) runs fall into each category at year ten. The first category would be unlucky and the third would be lucky.
Our Monte Carlo simulator calculates a sequence of returns for the entire 40-year period. Typically, we would expect just about any model to favor those that have grown well during the first ten years (the lucky portfolios) and to caution against those that have fallen (the unlucky portfolios). You can always make hypothetical models that do otherwise, but they are not likely to be reasonable.
We really do not have to look at valuations to support your observation that:
Quote:
"But I could imagine a possible result where a $1,000,000 portfolio fell to $800,000, but a 4 percent SWR rose to 5 percent...,
which would lead one to conclude that Sam is in a relatively similar position to where he started despite the unfavorable history."
We simply have to observe that portfolio balances vary above and below a nominal safe withdrawal rate pattern. Variations such as you have identified are quite common and they do not represent extreme cases that end in failure.
| Date: Wed May 21, 2003 From:gummy |
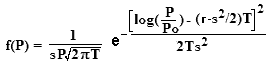
So I start with umpteen shares at the starting price (that's Po) and, each year (at T=1, 2, 3 ...),
sell sufficiently many shares at the current price (that's P) to meet my withdrawal requirements.
I then look at the resultant distribution of portfolios after, say, 10 years like so:

... to see how many survived.
Then I compare to the fraction of Monte Carlo simulations that would have survived.
Then I puff on my pipe for a while ... thinking ...
| Date: Wed May 21, 2003 From: WiseNLucky |
Since we are not discussing asset allocation here we must be assuming that AA stays static throughout this process, and that all portfolios have the same AA. That would never happen in real life but is necessary for mathematical purposes in our example, at least as I have read it.
Mr. MC is saying is that there is a 99% probability that Sam's portfolio, if withdrawals of 4% start on a specific date, will survive 40 years. That being said, if 1000 portfolios start on the same date as Sam's, and if all those portfolios have a 4% withdrawal rate, then all 1000 portfolios will have exactly the same result. They will all succeed or will all fail. There will not be 990 winners and 10 losers.
The only way to have 990 winners and 10 losers is to have portfolio withdrawal start on different dates. So, if Sally jumps on the bandwagon 10 years down the road with the same portfolio and the same withdrawal rate on that date as Sam, she will share the same result as Sam and all his other 999 fellows. And that result was set in stone the day they started withdrawal.
What am I missing here?
| Date: Wed May 21, 2003 From: JWR1945 |
Monte Carlo models generate a large number of future sequences of stock market returns that are possible. Inside of the model are introduced selected sources of randomness. They are restricted so that they have the same statistical characteristics as we have seen in the past. With care such models can match the entire statistical distribution that has been seen in the past. They are not limited to matching effects close to any extreme (e.g., high levels of safety). With care no single source of randomness is large enough to make much of a difference. The results from looking at a large number runs are well behaved. You can examine complex transactions such as annual re-balancing in terms of their statistical behavior.
When you refer to a specific date, you are talking about a single, possible future sequence of returns. All identical portfolios for that possible sequence have identical balances. When we refer to what happens ten years after the start of a 40-year period of withdrawals, we look at each possible sequence and examine its behavior at the end of year ten. We continue until we have looked at a large number of such sequences.
Please continue asking questions until we have clarified this issue and answered your question.
| Date: Wed May 21, 2003 From: KenM |
John R
May I (cautiously) suggest that issues such as whether it is a simple/gummy/raddr MC simulation or whether the portfolio starts at high or low market valuations are irrelevant to answering my basic question about MC simulations and probabilities of success/failure at the "theoretical, in principle" level. As I see it, an F1 racing car/a Mercedes/a Model T is a car is a car is a car. At the very basic level an MC model is a model is a model is a model. The mechanics are the same - input certain parameters; do, say, 1000 runs; if only 10 fail then you have 99% probability of success.
gummy
Question #1: What fraction of the jillion 10-year portfolios will survive another 30-years?
If one does MC simulations, then this Question might have an answer like 90% (adopting KenM's number. But we've assumed that 99% of the jillion portflios survive 40 years! So how come only 90% survive the last 30 years?
May I suggest (even more cautiously) that I would not put it in this way? At the start we have one portfolio with a zillion possible outcomes over the next 40 years (we can plot a zillion pretty pictures of graphs of portfolio value curves). 99% or 0.99zillion survive and 1% or 0.01zillion fail. However after 10 years, we look through the zillion graphs and find that we have to discard, say, 0.9zillion of the outcomes because our portfolio value is $P10 at that time, so we now have only 0.1zillion outcomes left. But, as we haven't failed yet, we still have the same 0.01zillion possible failures and successes must therefore be 0.09zillion. So our probability of survival after 10 years is now only 90% at a portfolio value of $P10. Or is mathematical life more complicated than that?
The best way I can think of the problem is as one of gummy's pretty pictures which I've come to love almost as much as the Sam/Sally format (I can sincerely say that without Sam/Sally I would still be very much lost in this problem - of course I may still be completely wrong so I may still be completely lost ). So, if I carry out 1000 MC runs starting with a portfolio value of $1million, 4% SWR, $40,000 withdrawal and 99% safety I get a graph of 1000 curves of portfolio value over 40 years, 990 will succeed, 10 will fail. After 10 years my portfolio value has gone up and down and is now $800,000 and I select the curve from the graph which best approximates the portfolio behaviour over the 10 years. Can I then run my finger along this curve and if it lasts the remaining 30 years say bingo I've now got 100% safety or 100% failure- don't be ridiculous KenM of course that's absurd - if you can do that you can predict the future from the past. So all I can say is that the history of my portfolio over the last 10 years is irrelevant to the possible future outcome over the next 30 years and I will have to carry out 1000 MC runs again starting at $800,000 and for 30 years i.e. superimposing another 1000 outcome curves on the graph with the origin at 10 years/$800,000. Which may show that if I maintained my current high withdrawal level at this low portfolio value I only have 90% safety. If I still want 99% safety then the SWR is, say, 4.5% and the withdrawal would have to be reduced to $36,000.
If I'm right ( I'm happy to be proved wrong) then I would be deluding myself to think that an initial SWR at a specified safety level would give me the same safety level throughout my whole retirement period.
John R
You are developing what I consider to be a great talent
My wife calls it being stubborn
However , I think I now see the relevance of gummy's inflation parameter and particularly of raddr's mean reversion in their MC models. If the portfolio value drops to $800,000 at 10 years then the market must have gone down substantially and mean recersion says it's more likely in future to go up rather than go down. (i.e. you can predict the future from the past ) and the safety level may not be as bad as it looks. But how can you assess the effect of that on future safety for the remaining 30 years? I think I might be happier with randomness a simpler method for my simple mind.
WiseNLucky - like your signature
Nice to see someone else admitting to being as confused as me
And that result was set in stone the day they started withdrawal.
What am I missing here?
I don't think that we're talking about 1000 portfolios but one portfolio with a zillion outcomes - Monte Carlo produces,say, 1000 outcomes for a 40 year period which are (hopefully) representative of those zillion. One of those zillion will be "set in stone" from the start date but which one?
Rereading all the above it looks like I definitely know what I'm talking about - but it's all very tentative - but I'm too lazy to retype.
| Date: Wed May 21, 2003 From: bpp |
Sam was given a 99% probability of success 10 years ago, but we don't know what his probability is now. It could be higher, or it could be lower. To take an extreme case, suppose Sally met Sam in Sam's 39th year of retirement, and they both plan to kick in one year. Sam has some portfolio balance, and some planned withdrawal based on MC studies made 39 years ago. Obviously, his probability of success is not 99% anymore. (In fact, just by looking at his portfolio balance he knows whether he will make it or not through that final sunset year.) And Sally, of course, with the same portfolio balance can choose to share Sam's fate by taking the same withdrawal. But the probability of success for the pair of them is obviously no longer 99%. Whether it is higher or lower (i.e., 100% or 0% in this case) would have to be looked at afresh given the current portfolio balance(s).
Back this argument out a few years, and one can see that at Sam's 10-year mark, Sally can guarantee that she shares Sam's fate by using the same withdrawal rate on the same-sized portfolio, but that their shared probability is no longer 99%. Whether it is higher or lower would have to be studied again using a 30-year MC instead of the original 40-year one.
Anyway, a random idea to kick around, for what it may be worth.
Cheers,
Bpp
| Date: Wed May 21, 2003 From: JWR1945 |
The highlighted portion is true and extremely important. The various approaches (including those using historical sequences as well as Monte Carlo models) start the first year assuming zero information that is directly based on valuations.
Continuing. KenM
Quote:
"However , I think I now see the relevance of gummy's inflation parameter and particularly of raddr's mean reversion in their MC
models. If the portfolio value drops to $800,000 at 10 years then the market must have gone down substantially and mean reversion
says it's more likely in future to go up rather than go down (i.e. you can predict the future from the past) and the safety level may not
be as bad as it looks. But how can you assess the effect of that on future safety for the remaining 30 years? I think I might be
happier with randomness a simpler method for my simple mind." [Emphasis added.]
There are ways to do this. With any of the models you look at all of the 40-year runs with balances close to $800K at the end of year 10. You then calculate the percentage of those particular runs that last for the remaining 30 years (and the percentage of those that fail). That gives you the conditional probability of success for that particular situation (e.g., a balance of $800K after ten years). The three different models will produce three different answers. From Gummy's model you will see the influence of ten years of inflation data. From raddr's model you will see the influence of ten years of mean reversion.
| Date: Wed May 21, 2003 From: KenM |
As I understand it, (and as usual I may be wrong) with a simple MC model the 99% safe SWR will be different for each year depending on the number of years remaining, i.e. at the start of 40 years the SWR is, say, 4% of starting portfolio value. At 10 years it might be, say, 4.5% of the 10 year current portfolio value, at 20 years 5%, etc, etc, until at the 39th year the SWR will be 100% of final portfolio value ( not sure about this bit, but presumably still only 99% safe until you get the money in the bank - remember the asteroids). So you could plot a curve of SWR's from 4% to 100% over the 40 year period. If your actual %withdrawal of current portfolio value in a particular year is equal to or less than that year's SWR then you are a minimum 99% safe. So Sally slots in to whichever year at the relevant SWR - as long as her asset allocation is the same as Sam's then her % safety is the same for the remainder of Sam's MC simulation period.
| Date: Wed May 21, 2003 From: JWR1945 |
With his example bpp has helped us understand conditional probabilities.
Have fun.
John R.
| Date: Wed May 21, 2003 From: KenM |
I may have missed it but I can't remember seeing descriptions of such analyses being undertaken - or perhaps I didn't understand what I was looking at at the time. Anywhere you suggest I can look?
| Date: Thu May 22, 2003 From: gummy |
If one assumes that annual returns have a predefined distribution (example: lognormal with prescribed Mean and Volatility), then looking at 1000 identical portfolios, all starting at the same time (experiencing different sequences of returns according to that distribution), is the same as looking at a single portfolio with 1000 possible futures (experiencing different sequences of returns according to that distribution). I guess Monte Carlo does the former to estimate the latter. The problem with looking at the various portfolios at the 10 year mark is that they ain't identical. Each has it's own withdrawal rate.
Nevertheless, the question remains, for me (and Ken?):
How are the 1000 starting portfolios (or a jillion) ... how are they doing at the 1-year mark or the 2-year or 3-year ... and what is the distribution of withdrawal rates and how does that compare to the MC "safe" rate at T=1, 2, 3 (assuming years left = 39 or 38 or 37 ...).
Anyway, the discussion on this board are so interesting that I'd like to put excerpts on my website.
Who should I ask for permission?
In order to see the evolution of withdrawal rates, survivors, etc. etc. (as time progresses) I've been playing with a spreadsheet which (so far) looks like this: picture.
My wife is happy. It keeps me out of her hair 