If we take the current P/E as 25 (roughly the current S&P 500 P/E, as I write this), and assume it varies inversely with the 10-year treasury, we get this historical (and fictitious) chart of earlier P/E ratios:

Compare to the actual historical P/E Ratios:

So, based upon historical Bond Rates, is the market over-priced?

>You're assuming a current P/E of 25? Why don't you determine what the P/E should be, today?
Okay, here's what we'll do:

1. Plot E/P (data obtained here) vs 10-year T-bond Rate.
2. Determine the best fit.
3. Use that (based upon data for the last thirty years or so) to determine what the current P/E ratio should be.

Here are the charts: The Best Fit to the E/P vs T-bond data is the straight line:       E/P = 0.9402 (T-bond) - 0.0048 Hence, we can get our P/E Ratio estimate from the Magic Formula:       P/E = 1/{0.9402 (T-bond) - 0.48%} Here, we put (T-bond) = 0.052 if the T-bond Rate is 5.2%. If we plot this for thirty years (actually Jan, 1970 to Dec, 2000) we get the following chart:

>So? So, the current P/E Ratio should be ... uh, let's see, the 10-year Treasury Bond Yield (obtained is 5.25% as of July 10, 2001 (that's today) so, from our Magic Formula, P/E = 1/{ 0.9402(0.0525) - 0.0048} = 22 >Exactly? Uh ... the Magic Formula gives: 22.4413999 >Hey! That's pretty close, isn't it? Pretty close.

>So, this was your idea, right? I mean, to consider historical long term bond ... Uh ... no. It was suggested by Don S.

>So, Greenspan uses historical E/P ratios to see if the market is overpriced?
Actually, I think, according to Yardeni, he uses 12-month-ahead earnings instead of our 12-trailing and ...

>So, why didn't you?
Uh ... wait'll I find the data ...

However, one could use the Estimated Earnings found here, but these often win prizes for the best fiction of the year. Just remember:

There once was an Index: S&P
Whose ratios evoked much glee
Pick two numbers at random
Divide them in tandem
And generate your own P-over-E

For example, we could do this

• If Fair Value P/E is 24, and
• Estimated Earnings are $35.47, then
• S&P 500 should be 24 * 35.47 = 851.28 by year's end, so
• a current S&P 500 of 917.93
• makes the current S&P overpriced by 917.93/851.28 - 1 = 0.78 or 7.8%

>I take it that this magic formula is valid back to the beginning of time ...
Well, actually ... uh ... it's pretty lousy prior to 1970. For example, from 1953 to 2000 the E/P vs 10-year T-bond looked like this:

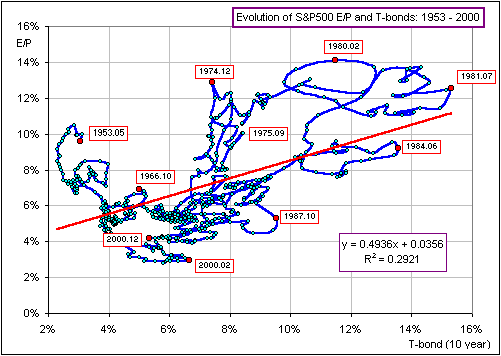
The goodness of the fit ...

>The goodness of the fit? Are we talking shoes or ...?
... is measured by R-squared (see R-squared bumpf) and you can see it's pretty lousy for this entire period. But it's interesting, eh? Look at the crash of 1987 where ...

>Am I supposed to be able to follow that blue line?
Why not?

>Anyway, what does this 1953-2000 goodness of fit predict for the current P/E?
Don't ask.

Here, you can play with the magic formula:       1/{0.9402 (T-bond) - 0.0048} based upon 1970-2000 S&P500 data: 10-year Treasury Rate =% "Fair Value" P/E = Get Bond Yields here.

Here, you can play with the magic formula:       1/{0.4936 (T-bond) + 0.0356} based upon 1953-2000 S&P500 data: 10-year Treasury Rate =% "Fair Value" P/E =

>That's quite a difference! I mean, what good is ...?
If you'd like to see whether looking at the past 10 years will say something about the next 10 years, take a gander at these charts which show the monthly oscillations of E/P versus the 10-year T-bonds ... and the "Best Fit" line:

>I don't think I'd like to use the past ten years to predict ...
... the next ten? I'm with you.

>So, how do these formulas compare to the actual P/E?

>Wow! The late 1990s were really out of whack!
Yes, and that has a name.
>Irrational Exuberance?
You got it!

'course, y'all may like to generate your own P?E ratio(s)

I was surprised to learn that some calculate not E/P values, but use the Average Earnings over the previous 10 years. Starting in 1970, this would give the following charts, comparing E/P and E10/P vs T-bonds:

Then again, Shiller uses the "real, CPI-adjusted" S&P500 and Earnings, assuming that CPI = 100 in Jan, 2000. That means that each S&P500 and Earnings value, for month M, is multiplied by CPI(Jan/00)/CPI(M). This gives:

Using :       1/{0.8552 (T-bond) + 0.0055} based upon 1970-2000 S&P500 data: 10-year Treasury Rate =% "Fair Value" P/E10 =

10 years ago, the yield on the 10-year Treasuries was 6% ... and the 30-year average ain't bad

and with an 80% correlation P/E5 ain't bad either ! Using :       1/{0.8313 (T-bond) - 0.0043} based upon 1970-2000 S&P500 data: 10-year Treasury Rate =% "Fair Value" P/E5 =

See also updated info