from the Fund Library
Date: 07-Jan-98 - 12:00 PM
Subject: Regression to the Mean (?)
From: gummy
|
Once-upon-a-time and one-of-these-days I promised to look at this.
I don't have PalTrak so (at Bylo's suggestion) I downloaded the Demo Version,
then I
- Extracted all the Cdn Equity funds for which there was a history
of annual returns from July/87 to July/97 (80 of 'em)
- For each year
(ending in July/87, July/88, ... to July/97) I calculated the Mean Annual Return of all
80 funds.
- For each fund, I determined its deviation from the (above)
Mean Annual Return (for the years mentioned).
- I plotted these deviations for the
Best Fund of 1987.
This is what I got:
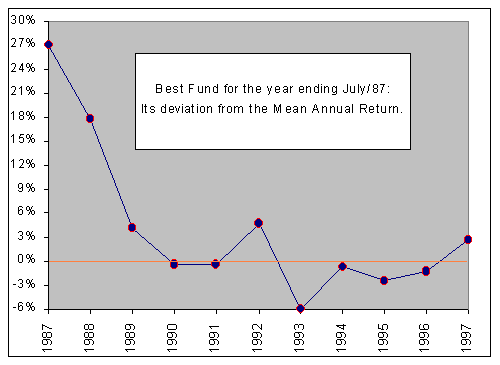
Is this Regression to the Mean or is it just that this fund had an unusually good
first year? I dunno.
Then I plotted the deviation for the worst fund:
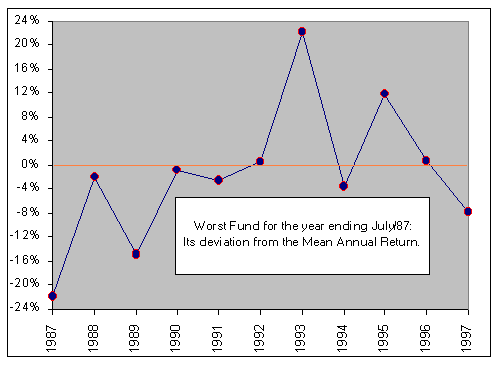
Is this Regression to the Mean or is it just
that this fund had an unusually bad first year? I dunno.
Then, the deviation for the
"median" fund: in July/87, this fund stood 40th in the collection of 80 funds.
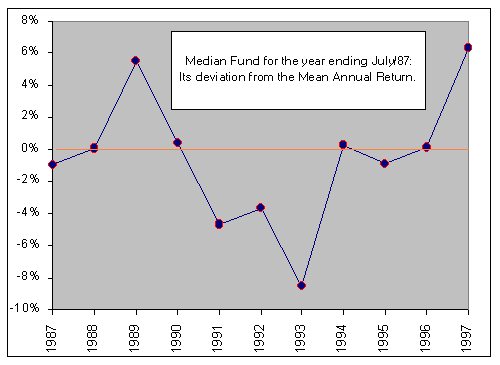
Finally, I plotted the deviation for all the first
quartile funds (as of July/87). That is, for each year, I took the mean of this group of
funds and calculated the deviation of this mean to the mean of all 80 funds.
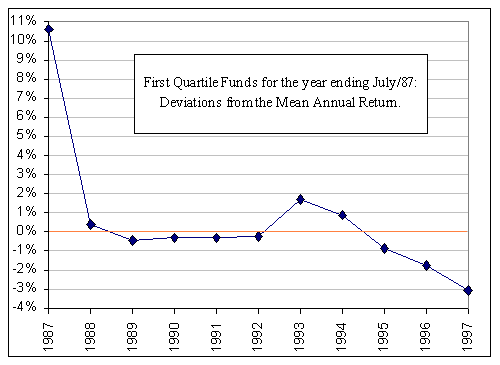
Finally, finally, I did the same for the 4th
quartile funds:
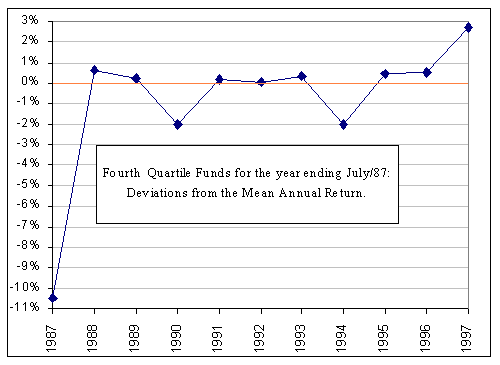
Conclusions?
You decide.
Date: 07-Jan-98 - 8:23 PM
Subject: RE: Regression to the Mean (?)
From: thbox
|
gummy:
These are VERY nice pictures, and I appreciate your industry. Regarding the "median" and indeed the "first quartile" groups over the time frame, it surprises me not at all that the group approaches the group average. Regarding the "best" and "worst", I don't think it proves much, since they're "best" and "worst" only in the year preceding the start date. IN these cases, I think it would be better to take a fund with a history of out (or under) performance over a five (or ten) year period, and THEN evaluate whether it could maintain that over the next period. I would still be interested if you could find some mathematical and/or theoretical justification for the hypothesis.
By the way, the original thread was How does MER affect the performance of a fund, and the date of our exchange was (around) 26.12.97
Thanks
Date: 08-Jan-98 - 8:38 AM
Subject: RE: Regression to the Mean (?)
From: gummy
|
thbox: the theoretical justification assumes the annual returns are stochastic and normally distributed with a (meaningful) standard deviation.
If you consider a "first quartile group" of random variables (in 1987) , some of them will be there simply because of their (normally distributed) deviations from the mean. Later (in 1997), these deviants will be closer to the mean. (It is highly unlikely that the random deviations from the mean will continue to be in the same direction.) This results in a "Regression to the Mean".
The question really is: Do the annual returns of mutual funds & stocks satisfy the assumptions of randomness required for "Regression to the Mean"?
Many (most?) financial analysts think so (else they wouldn't place so much stock* in statistical paraphernalia such as Standard Deviation, Regression, etc.)
{* sorry}
You read such sloppy statements as "2/3 of monthly returns will lie within one standard deviation of the mean". I've tried to validate (invalidate?) this particular claim and find that (historically) it's often more like 75%, sometimes 80%.
The trouble with Mathies is that there are so many neat formulas to apply, relationships to enjoy, theorems to prove ... if only they can make certain assumptions about the data ... so they do.
Often, the "best" of anything is defined as the guy that minimized the "Root-Mean-Square" error (error = deviation).
The "best" straight line fit to data (that's the "regression line")
... the "best" polynomial approximation to data.
These minimize the RMS deviation.
Why minimize the RMS deviation?
Why not minimize the LARGEST deviation? Surely one would be more comfortable with the guarantee:
the LARGEST error is no more than 1%
instead of
the RMS error is no more than 1%.
The RMS deviation is minimized because there are absolutely breathtaking formulas/relationships/theorems available for RMS deviation.
You wouldn't believe how excited Mathies get when they see a beautiful formula.
(For example, perhaps the three sexiest numbers in the universe are i = SQRT(-1) and π and e and, would-you-believe, eiπ = -1. Now that is one beeyutiful formula!!)
Aah, but I digress.
My goal is to investigate the underlying assumptions ... hence the pretty pictures.
Date: 08-Jan-98 - 8:53 AM
Subject: RE: Regression to the Mean (?)
From: Bylo
|
I can't resist...
I used to think math was no fun,
'Cause I couldn't see how it was done.
Now Euler's my hero,
for I now see why 0
= eiπ + 1.
The was a young student of Trinity
who computed the square of infinity.
but it gave him the fidgets
to write down the digits,
so he chucked it and took up divinity.
Credit: Found somewhere in cyberspace.
Date: 08-Jan-98 - 9:16 PM
Subject: RE: Regression to the Mean (?)
From: Still Stupid But
|
Huh??
Math teachers! ALways with that crazy language I can't understand. Dang blast 'em anyhows!
Date: 09-Jan-98 - 12:23 AM
Subject: RE: Regression to the Mean (?)
From: Clumpy
|
Humour from Cyberspace
A statistician can have his head in a pizza oven and his feet in a bucket of ice, and he will tell you that on the average he feels fine.
Date: 04-Feb-98 - 8:00 AM
Subject: RE: Regression to the Mean (?)
From: Bylo
|
Y'all might wanna read this Reversion to the Mean: Sir Isaac Newton Comes to Wall Street by John Bogle -- mah second mose-fay-vo-rite invessmint wrahter.
Date: 04-Feb-98 - 8:14 AM
Subject: RE: Regression to the Mean (?)
From: Bylo
|
An here's wha mah furst mose-fay-vo-rite invessmint wrahter (an all-roun' good-ole-boy) is up to raht naw Berkshire owns large stake of world silver supply. Yesterday BRK.A closed at US$52,600 (yup, a share!).
Golly gee...
Date: 04-Feb-98 - 12:32 PM
Subject: RE: Regression to the Mean (?)
From: PK
|
To Gummy:
I hope you take this note in the spirit in which it is intended -- intellectual debate. I know in a posting like this it is difficult to capture the complete depth of your understanding, so if I misinterpret some of your statements I apologize in advance.
It seems to me you do not understand the concept of regression to the mean and as heretical as it might sound I don't think Mr. Bogel gets it either. You both seem to be confusing what you think you see with what actually occurs and you don't take probabilies into consideration.
First, regression to the mean does not occur with randomly occuring variables. Also financial theorists who believe in effecient markets do not believe in regression to the mean (RM).
I'll start with a slight digression. The efficient market theory is not one theory but a continum of beliefs. Almost all theorists believe in the weak form (ie- historic return patterns have zero predictive value or to state another way chartists are about as useful as astrologists). At the next level are believers in the semi-strong form (ie. all publically available information is reflected in the price of securities). The evidence for this is not clear cut but most theorists believe it comes pretty close to the truth. Finally there are believers in the strong form (ie both public and privately available info is reflected in security prices). Almost no one believes this.
If we therefore focus on semi-strong beliefs, the conclusion is that excess returns can not be achieved with any consistency by examining publically available info. This is the exact opposite of believing in regression to the mean. If you believed in RM you could structure an investment strategy to take advantage of the phenomenon. You could sell short 1st quartile performers and/or buy long 4th quartile performers. In some ways this is akin to a value strategy. The flip side of this type of serial dependence would be momentum investors (ie trends tend to linger so don't "fight the tape").
The problem with Mr. Bogel's analysis is he fails to consider probabilities. Take as an example a truely random test -- rolling dice that are fair. If you roll dice, say 10,000 times you would expect to get about 833 twelves. Let's call these 1st quartile performers (after all they rolled 12!). In the next go around these 833 rollers of 12 would be expected to roll a normal distribution of results and their mean roll would be about 7. But this does not demonstrate regression to the mean. If RM did occur here you would expect to find a higher percentage of 7 rolls then you would otherwise. In other words the result of the 2nd roll is independent of the result of the first roll. With RM you are assuming serial dependency. So while the mean result of the 2nd roll does become 7, you would expect this probabilistically.
So, just because it looks like RM occurs does not mean that it really occurs when probabilities are considered.
Just one more digression that you might find interesting. There are some effecient market proponents who are currently examining the opposite of RM -- ie. trends tend to linger, at least for a short time. The results to date are inclusive but people like nobel prize winner William Sharpe are interested enough in the data to examine it in more detail. The premise is that 1st quartile performers tend to slightly outperform the mean in the next period. The jury is out on this one but if you look at your first chart this result did occur.
Date: 04-Feb-98 - 7:34 PM
Subject: RE: Regression to the Mean (?)
From: gummy
|
PK: good points. My intent was NOT to illustrate Regression to the Mean, but rather to follow a fund (or funds) and see whether this common usage (among many investment gurus) is justified. One often reads that Regression to the Mean will eventually reduce this higher-flyer to mediocrity. Not only do I look with scepticism upon the RM statements, but also any statements which treat Stock/MutualFund prices as random variables with normally distributed deviations from their mean, such as (and this I really find amusing): 2/3 of returns will (that's future tense) lie within one SD of the mean.
Date: 04-Feb-98 - 8:02 PM
Subject: RE: Regression to the Mean (?)
From: rge
|
I can't help but make two observations:
1. Figures can't lie, but liars can figure!
2. There are Lies, DAMN LIES and STATISTICS
I don't understand what it all means, Gummy, but I am impressed at your computational skills and your ability to post such impressive graphs on these threads. Keep up the Good Work for the sake of those more mathematically literate (numerate?) that me.
Date: 04-Feb-98 - 8:15 PM
Subject: RE: Regression to the Mean (?)
From: Tim
|
Gummy, it's been about 12 years since my last statistics course (and even then it was in one ear and out the other!) Others in this thread are nit-picking (for the lack of a better word) the statistical validity of your claims, but I think they are missing the broad point that your pretty pictures are illustrating: mutual fund managers cannot, over the long term, significantly outperform.
Date: 04-Feb-98 - 8:18 PM
Subject: RE: Regression to the Mean (?)
From: Tim
|
Gummy, it's been about 12 years since my last statistics course (and even then it was in one ear and out the other!) Others in this thread are nit-picking (for the lack of a better word) the statistical validity of your claims, but I think they are missing the broad point that your pretty pictures are illustrating: mutual fund managers cannot, over the long term, significantly outperform.
By the way, thanks for the effort you put into these threads - much appreciated!
Date: 04-Feb-98 - 11:29 PM
Subject: RE: Regression to the Mean (?)
From: jd
|
Not only do I look with scepticism upon the RM statements, but also any statements which treat Stock/MutualFund prices as random variables with normally distributed deviations from their mean, such as (and this I really find amusing): 2/3 of returns will (that's future tense) lie within one SD of the mean.
Lighten up Gumby, it's an approximation of reality. Not perfect, of course, but it is responsible for a lot of the financial models that both you and I - explicitly or implicitly - base our investment decisions on.
Although this may seem a paradox, all science is dominated by the idea of approximation.
Bertrand Russell
Date: 05-Feb-98 - 12:18 AM
Subject: RE: Regression to the Mean (?)
From: PK
|
To Gummy
I appreciate your clarification concerning your opinion of regression to the mean -- it seems I misunderstood your position and we share skeptism concerning RM.
Re your comments about 2/3 etc., most financial analysts worth listening to would not make such a definitive comment. You usually here them say that the distributions of returns are close enough to normality to allow for analysis. You'll also find that most research has found that returns tend to be positively skewed and that there exists more extreme results than you would expect with perferctly normal distributions (this later finding goes a long way in explaining your observation that more than 2/3 of results are found within 1 standard deviation unit of the mean).
I've often observed that when you here people making really definitive statements they usually don't understand what their taking about (or at least as much as they think they do). So the next time you here someone say 2/3s of returns will occur within 1 SD, your entitled to laugh -- but remember that people who do understand the stats would not make such a blanket statement.
Date: 05-Feb-98 - 8:43 AM
Subject: RE: Regression to the Mean (?)
From: Gumby
|
jd: let me browse a bit 'n find statements which imply, to the novice, that these are facts (read "gospel"), based upon sophisticated mathematical gesticulations of no concern (read "understanding") to the great-unwashed.
Now, if they said approximations to the real world ...
P.S. I spent 30 years as an applied math prof and shouting "approximation to the real world" was something I became very good at.
Date: 05-Feb-98 - 8:47 AM
Subject: RE: Regression to the Mean (?)
From: gummy
|
I have this theory (yet-to-be-verified) that the height of six foot males will regress to the mean. Once validated, I expect to gain a couple of inches.
Date: 05-Feb-98 - 9:23 AM
Subject: RE: Regression to the Mean (?)
From: Learner
|
Isn't there a story about how :
A scientist thinks the real world is an approximation to his equations:
An engineer thinks his equations approximate the real world:
A computer scientist keeps tweaking 'til he gets the answer he wants;
Can anyone fill in the blanks for a FP? Sorry folks I couldn't resist.
Date: 05-Feb-98 - 10:15 AM
Subject: RE: Regression to the Mean (?)
From: PK
|
To Gummy -
I think I read somewhere that the very first person to illustrate RM did so in a study of male height. The sons of tall fathers were found to be ,on average, taller than the overall population but shorter than the average of tall father's height -- Therefore RM
Date: 05-Feb-98 - 10:36 AM
Subject: RE: Regression to the Mean (?)
From: jd
|
"Regression was introduced by Francis Galton. In a famous paper1, Galton found that, although there was a tendency for tall parents ot have tall children and for short parents to have short childern, the average height of children of both parents of a given height tended to move or "regress" toward the average height in the population as a whole. Galton's law of universal regression was confirmed by his friend Karl Pearson, who collected more than a thousand records of heights of members of family groups2. He found that the average height of sons of a group of tall fathers was less than their fathers' height and the average height of sons of a group of short fathers was greater than their fathers' height, thus "regressing tall and shorts sons alike toward the average height of all men. In the words of Galton, this was "regression to mediocrity."
The modern interpretation is, however, quite different from Galton's view.
1. Francis Galton, "Family Likeness in Stature, " Proceedings of the Royal Society, London, vol 40, 1886, pp.42-72.
2. K. Pearson and A. Lee, "On the Laws of Inheritance," Biometrika, vol 2, Nov 1903, pp.357-462.
Date: 05-Feb-98 - 1:44 PM
Subject: RE: Regression to the Mean (?)
From: gummy
|
Aah ... found some references:
Here's a well-known and highly respected analyst:
Thus there are roughly two chances out of three that the outcome will lie within ...one standard deviation of the mean.
followed by
If the return can be assumed to be normally-distributed, this means that there are roughly two chances out of three that the actual return will lie between ...
Note the use of the word "If". Good for her (him?).
However, there's also:
What does the standard deviation number actually mean? It means that two thirds of the time the annual return of the asset will lie between 1 standard deviation above and 1 standard deviation below the mean value.
Note the use of the word "will". Bad for him (her?).
(I'd give you the URL but ... uh, there's this wee sign at the bottom which sez: "do not copy, distribute, reproduce, etc. etc." so don't tell nobody I stole these phrases. If you really want the references, e-mail me.)
Here's a (typical?) highly sophisticated (cough) theoretical analysis:
You want to increase your portfolio from $0 to $1,000,000 in 30 years (360 months, from Dec 31/67 to Dec 31/97).
You assume a 9% return on your investment.
You insist that your portfolio increase by a fixed amount each month
(a form of Value Averaging, I guess).
That's $1,000,000/360 = $2778 per month.
How much must you contribute each month?
Answer? You start with a $2778 contribution and either contribute or withdraw funds so as to maintain the $2778/month portfolio growth.
At 9% annual growth (as it turns out), you'll never need more than $2778 each month.
Now, let's get real-world:
Taking the actual TSE monthly returns (thank you, Jay), this is what you get:
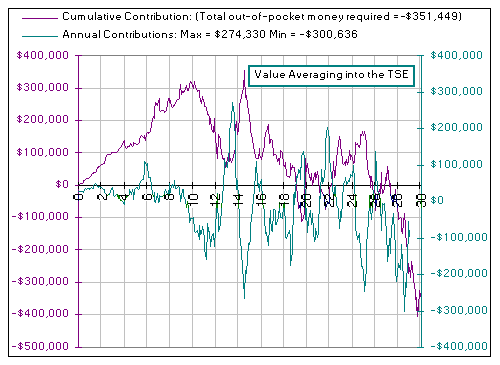
Although you end up taking piles of money from your portfolio (to maintain the $2778/month portfolio increase), you'll have to come up with $274,330 in one 12 month period (a year which includes the '87 crash).
Aah, ain't theoretical analysis wunnerful!
Date: 05-Feb-98 - 7:36 PM
Subject: RE: Regression to the Mean (?)
From: Bylo
|
And if yer still hankerin for more on RTM check out this discussion thread Bogle and Regression to the Mean, and other worrisome things.
Date: 05-Feb-98 - 7:52 PM
Subject: RE: Regression to the Mean (?)
From: rge
|
I have noticed on more than one thread that as the discussion progressed, the contributors regressed and became more mean. Am I beginning to understand this applied mathematics stuff?





