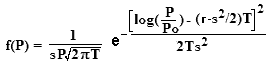So the suggestion (after looking carefully at data over the last umpteen years) is that the probability that a stock price will decrease after T years is roughly 1/T.

>Huh?
After 5 years, the probability that the stock price will decrease is (about) 20% ... and that's 1/5, expressed as a percentage.
After 10 years, the probability is (about) 10% and after ...

>Yeah, so is it true?
The calculations were based upon historical data, but I thought it'd be neat to see if good ol' Ito agreed.
Remember Ito?

>No.

He's got this neat formula for the distribution of stock prices after T years, starting at a price Po, assuming a Mean Return = r and Standard Deviation = s. See ? Note that P(T) is the price at time T so the question is:      "What's the probability that P / Po < 1?"

>And what's the answer?
'course, it'll depend upon our choice of r and s, but it's quite neat and ...

>A picture is worth a thousand ...
Here's a picture:

   

Note that, as T increases, one would expect the distribution of prices to be more widespread, more smeared, more spread out, more ... >More smeared? Is that a technical term? It's sorta like this For the chosen parameters, the Expected price gets bigger and the percentage of prices that lie below the starting price ... that gets smaller. >And all this is guaranteed ... by the Math, right? Guaranteed? Nothing is guaranteed! >What about death and taxes? Uh ... well, almost nothing is guaranteed.

for Part II